Nigel Hussey is a professor of physics at Radboud University and former director of the High Field Magnet Laboratory, both in Nijmegen, the Netherlands. Earlier this year Hussey was awarded a 2.5 million-euro grant from the European Research Council to study the flow of charge in exotic metals and superconductors under extreme conditions.
Photo credit: Gideon Laureijs
When a new material is synthesized, one of the first things scientists do is attach four wires to its surface, cool it down and measure its electrical resistance as a function of temperature. Plotting the data on a graph then tells these scientists how well the electrons flow through the material — in other words, whether it is an insulator, a semiconductor, a metal or even, at lower temperatures, a superconductor (a special class of conductor through which the electrons encounter zero resistance). Each of these yields its own telltale line on a graph.

Yet while this is one of the first physical properties we measure, it is very often the last to be understood.
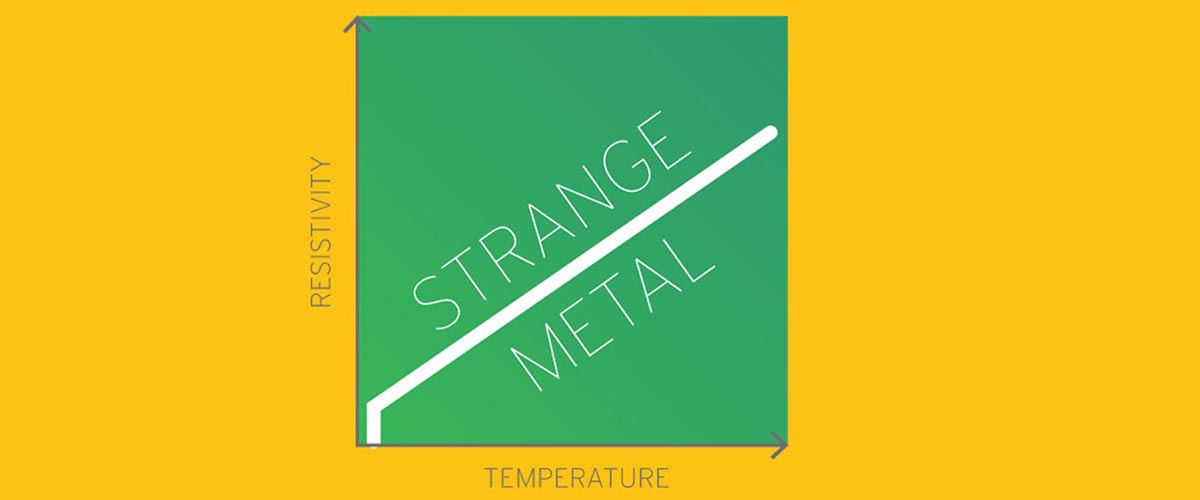
Never was this more the case than in an exclusive club of materials known as strange metals. True to their name, these materials exhibit behavior that condensed matter physicists like me (who study solid materials) find nothing short of spectacular. The graph representing that data is equally spectacular, depicting a line rising straight as an arrow across the page that telegraphs an entirely new, mysterious kind of physics at work — one we suspect is as elegantly simple as the line itself.
This behavior is all the more exciting because the strange metals club includes some very promising materials, such as cuprates and magic-angle twisted bilayer graphene. Both of these special materials superconduct at temperatures far higher than the conventional theory predicts, and it is hoped these "high-temperature superconductors" will ultimately revolutionize superconducting technology.
So, what makes that straight line so special? After all, electrical resistance in ordinary metals, like copper or platinum, also varies linearly with temperature. Indeed, this linearity is the precise reason why platinum is used as a thermometer standard.
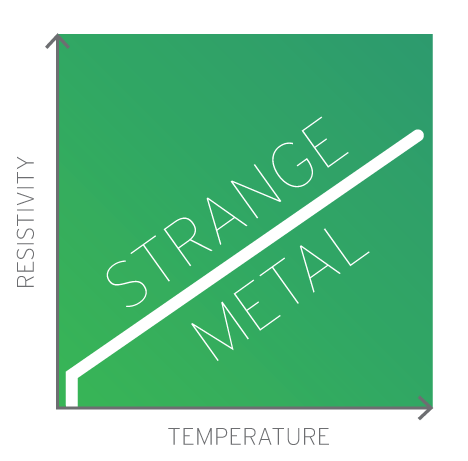
The thing is, normal metals exhibit this linearity only within a limited temperature range, as shown in the blue region of the line below. In the case of platinum, it’s between zero and a few hundred degrees Celsius. But if we were able to track the resistance over a much wider range of temperature, we would find that this linearity is only one of a host of different behaviors. The shape below shows this, reflecting how the resistance evolves at the quantum level.
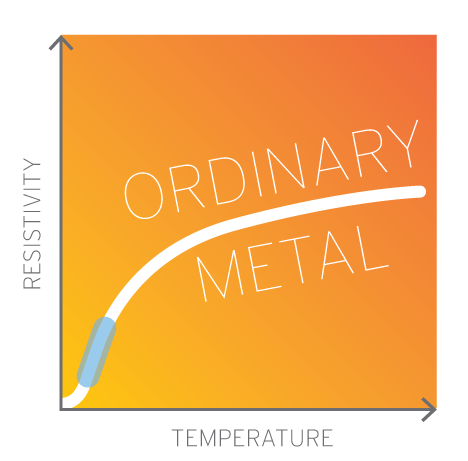
In normal metals, resistance happens because electrons collide with the vibrating atoms in their path, careering off course. The warmer it is, the more the atoms vibrate, and the more often electrons bump into them and scatter. This scattering grows linearly with temperature over an intermediate temperature range.
But when the metal gets extremely cold, there are fewer vibrations and less scattering. So the relationship between temperature and resistance becomes weaker and eventually levels off. There’s a change in behavior at the opposite end of the thermometer scale too. As ordinary metals warm up, their resistance eventually reaches a saturation value at which scattering ceases to increase.
So, in ordinary metals, the process that drives the resistance changes several times between absolute zero and its melting point. These individual processes, described in great detail in modern physics textbooks, are reflected in the curvy line on the orange graph.
Not so in the strange metals! Their resistance continues to decrease linearly with temperature (in some cases down to near absolute zero, where atoms are essentially frozen). And it increases linearly at temperatures far higher than is seen in ordinary metals, reaching values that are way beyond the conventional limit. At first glance, this might imply that electrons are being scattered multiple times between the atoms, violating a fundamental principle of physics. Optical measurements show, however, that this resistance at high temperature must be driven by some other, still unknown process that, in the end, might have nothing to do with the scattering of electrons.
What is most remarkable, and totally unexplained, is that across these very distinct temperature regimes, the resistance follows the same perfectly straight line. Such a relentless, unbounded growth in, say, the share price or the profits forecast of a registered company would look extremely attractive to a potential investor. To a scientist like me, such simplicity of form is also a thing of beauty, particularly when it continues to defy all conventional notions!
The plot (line) thickens
Now the plot thickens, thanks to high magnetic fields. Strange metals also exhibit a convention-defying linearity in another measurement: magnetoresistance.
First, let’s look at ordinary metals. When you put a normal metal in a magnetic field, the electrons experience a sideways force (called the Lorentz force) that causes them to follow a helical, rather than straight, trajectory, thereby increasing the material’s resistance. This is magnetoresistance, which in ordinary metals grows as the square of the magnetic field strength. The longer an electron travels before being scattered, the longer its curved trajectory relative to its original (straight) path, and the larger the magnetoresistance. So as the metal is cooled and its resistance drops, its magnetoresistance (for the same field strength) grows. The data look like this:
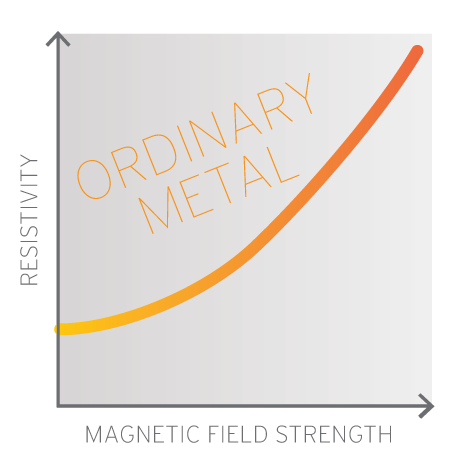
In strange metals, however, the magnetoresistance varies linearly with field strength over a very wide window. In some reported cases, this linearity reaches up to the very high field of 90 teslas, yielding once again an elegant straight line.
What's more, its slope is independent of temperature. This constancy suggests that magnetoresistance in strange metals, like their resistance in the absence of field, has nothing to do with the scattering we see in normal metals.
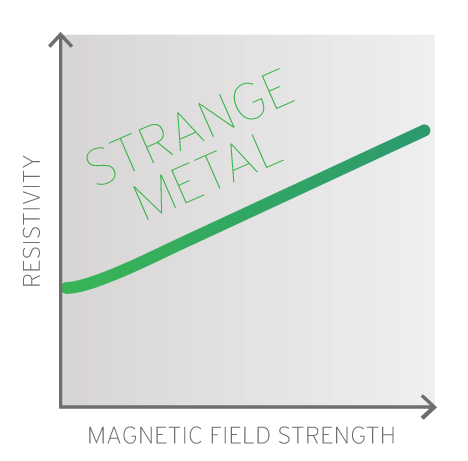
So in strange metals, the straight-line response in both field and temperature clearly points to something far deeper.
Finding out what that something is will ultimately require a radically new approach to our understanding of metallic behavior. I am among a number of researchers around the globe who are working hard to reconcile these two profound observations within such a single, unifying model. I consider this my greatest challenge as a condensed matter physicist. Such a model, once accepted, will surely herald a new chapter in the study of condensed matter.
While the bottom line to linearity remains to be discovered, one thing is certain: Given that many strange metals are also high-temperature superconductors, the rewards for developing such a model may extend far beyond a simple revision of our textbooks.
Story by Nigel Hussey